By looking at structures in nature there is a tendency to form a regular 2D or 3D grid from basic shapes. Going back from small scale into a large scale by slight “mistake“ in structure of the grid the whole shape or volume of the object is becoming irregular.
In the beginning of the project I started to look at several Platonic and Archimedean solids by joining them together and exploring the characteristics and composition of volumes (combining cuboctahedron and cube into aggregation the composition had some rhythmic and static behavior while combining icosahedron the volume and the shape was mostly irregular and unpredictable). Therefore for further development of the project I have chosen icosahedron as lattice structure for the research of the geometry inside by individually defined rules.
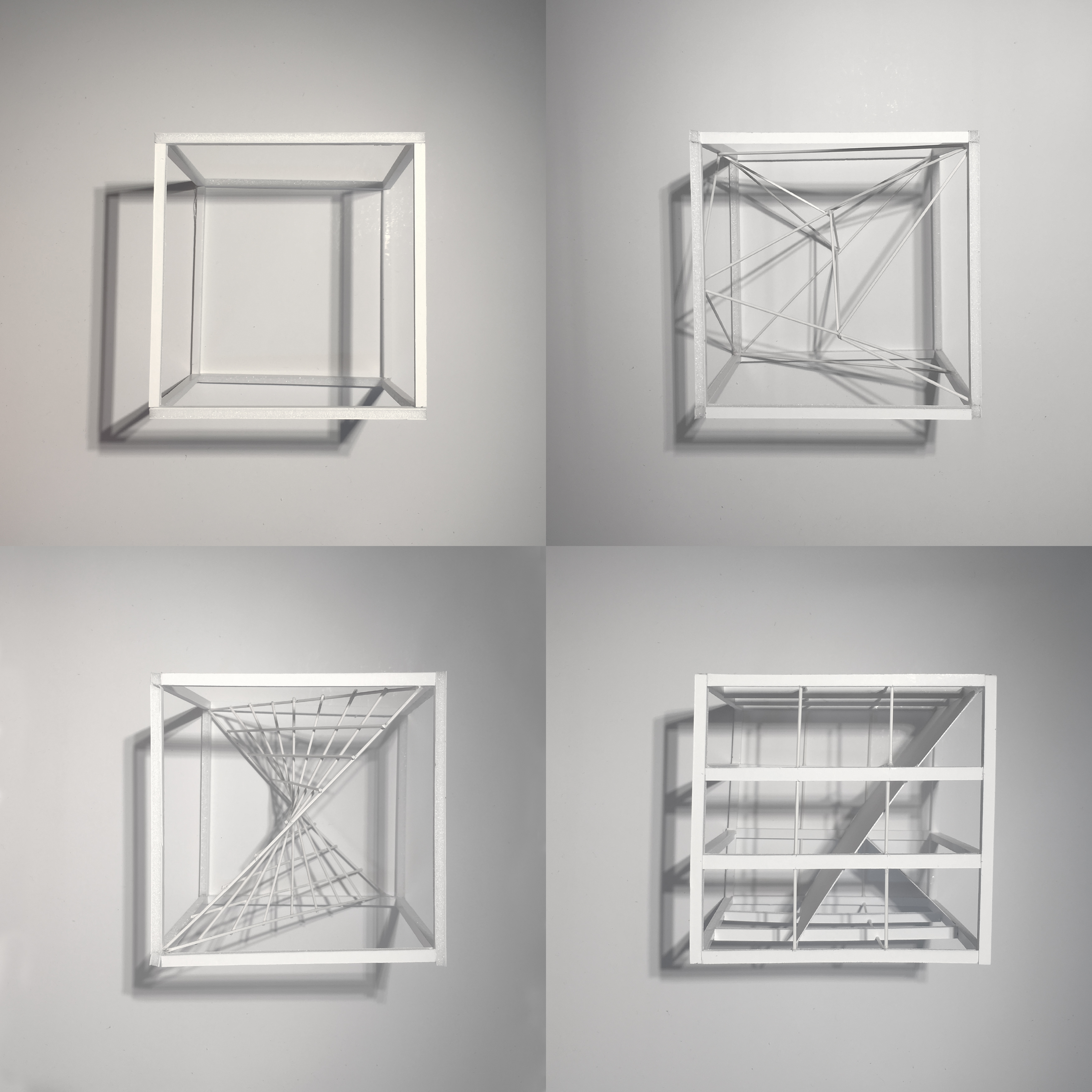
Some fragmented pictures of final physical model:


Grasshopper definition:
1 step – icosahedron geometry from weaverbird icosahedron:

2 step – designing a detail inside the icosahedron frame using kangaroo relaxation:

3 step – creating aggregation of icosahedron lattice structure using fox plugin:

Posters of project:
Thanks to tutors and guest critics for accurate comments! And it was fun (not easy at all) to make first steps in the new field of unknown.